the level 2 trigger

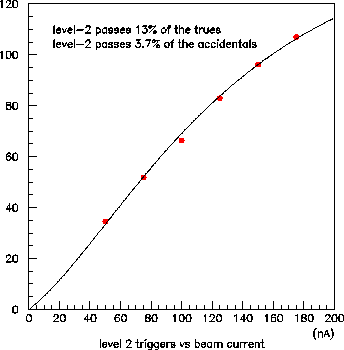
Figure 11: Level 2 scaler rate vs. beam current measured at the end of the June run period.

The level 2 trigger processor makes a fast estimate of the total energy in the lead glass wall and applies a threshold cut on this quantity. Presumably the signal events are more likely to contain a large neutral dose than nonsignal events. It is described within the model by the following formula,

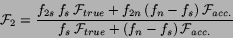$${\cal F}_2 = \frac{ f_{2s}\,f_s\,{\cal F}_{true} + f_{2n}\,(... ..._{acc.} }{ f_s\,{\cal F}_{true} + (f_n-f_s)\,{\cal F}_{acc.} }$$ (14)

where the input parameters $f_{2s}$ and $f_{2n}$ are the level 2 pass fractions for level 0 triggers (noCP events) that are signal (i.e. trues) and nonsignal (i.e. accidentals) respectively. The data overlayed with the model fit are shown in Fig. 11 for the scan taken at the end of the June period. If the level 2 processor is doing its job then one would expect that $f_{2s}\gg f_{2n}$ which is what is observed.